

The shape will still tessellate, so go ahead and fill up your paper.Then move it the same way you moved the squiggle (translate or rotate) so that the squiggle fits in exactly where you cut it out. On a large piece of paper, trace around your tile. Tape the squiggle into its new location.It’s important that the cut-out lines up along the new edge in the same place that it appeared on its original edge.You can either translate it straight across or rotate it.
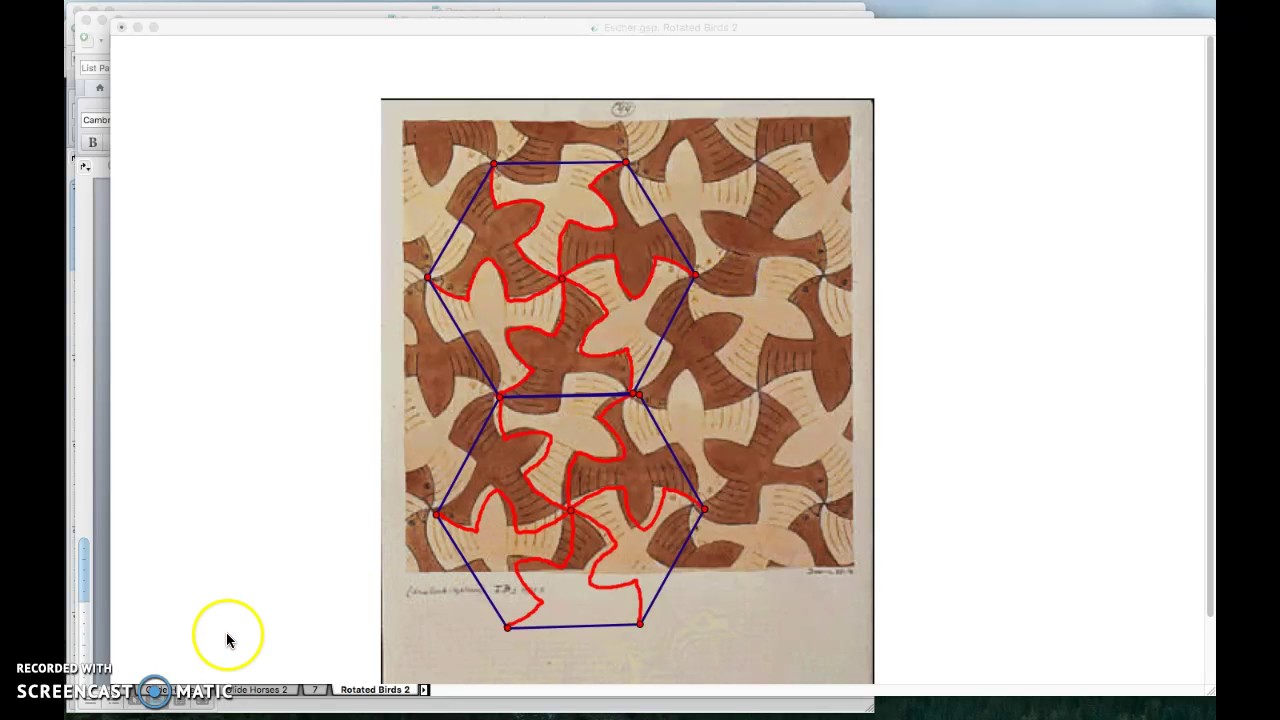
Cut out the squiggle, and move it to another side of your shape.Draw a “squiggle” on one side of your basic tile.The first time you do this, it’s easiest to start with a simple shape that you know will tessellate, like an equilateral triangle, a square, or a regular hexagon. Here’s how you can create your own Escher-like drawings. Tessellations are often called tilings, and that’s what you should think about: If I had tiles made in this shape, could I use them to tile my kitchen floor? Or would it be impossible? The first two tessellations above were made with a single geometric shape (called a tile) designed so that they can fit together without gaps or overlaps.
#Rotating tessellation how to#
So we’ll focus on how to make symmetric tessellations. It’s actually much harder to come up with these “aperiodic” tessellations than to come up with ones that have translational symmetry. The Penrose tiling shown below does not have any translational symmetry.

Many tessellations have translational symmetry, but it’s not strictly necessary. The idea is that the design could be continued infinitely far to cover the whole plane (though of course we can only draw a small portion of it). \( \newcommand\)Ī tessellation is a design using one ore more geometric shapes with no overlaps and no gaps.


 0 kommentar(er)
0 kommentar(er)
